Return

The Logic of Galilean and Newtonian Physics (space, time, simultaneity)
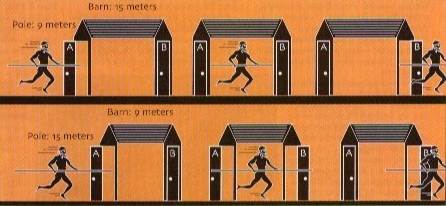
     Space and time are fundamental elements of Galilean and Newtonian physics, but they do not play fundamental roles in the new physics of relativity theory and quantum mechanics theory. These theories are interpretations of observed phenomena which depart from the logic of Galilean and Newtonian physics. The following figure, which appeared in the November 9, 2001 issue of Science (pg 1265), is an example of relativistic phenomena leading to the conclusion that absolute distance and time do not exist. The top and bottom parts of the figure show a runner with a pole moving through a barn with an observed relative velocity of .8 c. Each barn door is open only when the pole is passing through the doorway. The top part of the figure shows that in the reference frame of the barn, an observer(c) will determine that the runner is carrying a 9 meter pole through a 15 meter long barn, and that the barn doors are both closed when the runner is midway through the barn. The bottom part of the figure shows that in the reference frame of the runner, an observer(c) will determine that the runner is carrying a 15 meter pole through a 9 meter long barn, and that the barn doors are both open when the runner is midway through the barn.
     Lacking reasons for the phenomena represented by the above figure, it is understandable how one could conclude that we live in a universe in which absolute time and distance have no place. It is also understandable how others could hesitate to make this conclusion because history provides many examples of illogical conclusions being drawn from perplexing observed phenomena.
     According to relativity theory, when the runner in the above figure is midway through the barn, the doors are neither simultaneously open nor simultaneously closed (i.e. not as shown in either part of the above figure). Simultaneity, like absolute time and distance, does not exist according to relativity theory.
     The reader might consider that simultaneity does occur in nature as follows. Let event (e) be known as the event which occurs when the reader reads the following word and letter, event (e). Had a signal pulse having infinite speed been radiated in all directions from event (e), all other events in the universe that were occurring when the signal was received would be part of a set (E) of simultaneous events which includes event (e). The same set of events would occur and would be simultaneous even if the infinitely fast signal was not sent, and even if there was no infinitely fast signal. Is it not reasonable to conclude that simultaneity of events occurs in nature, even though it is not observed via an infinitely fast signal?
     If the quantum medium view is in accord with nature, the simultaneity of events can be observed (e.g. observers can determine the absolute distances to events and can determine the relative speeds of light arriving from the events, as discussed in the section, "Agreement among observers ...").

Return